Adjacent angles: The angles that do not overlap but share a common arm (side) and vertex are known as adjacent angles. When two rays meet at a similar endpoint, an angle is created, and neighboring angles are those that are always positioned next to one another. Two neighboring angles are referred to as linear pairs when their sum equals 180°. Let’s examine some examples of adjacent angles on this page and learn more about them.

About

There are two distinct kinds of angle pairs: adjacent angle and vertical angle. The fundamental idea of geometry, which we were taught in Classes 4 and 5, is these angle. The fundamental idea that is explored in academics is angles. Angles come in several varieties that we are taught in school.

The angle generated by a ray between its initial and final positions is the measure of the ray’s rotation when it is rotated about its terminus. When two rays are joined end to end, they produce an angle.

Two angle are sometimes used in geometry. Angle pairings can be of many different types, including supplemental, complementary, neighboring, linear, opposite, and so forth. This article will go into great detail about the definitions of vertical and neighboring angle.

Adjacent Angles: What Are They?

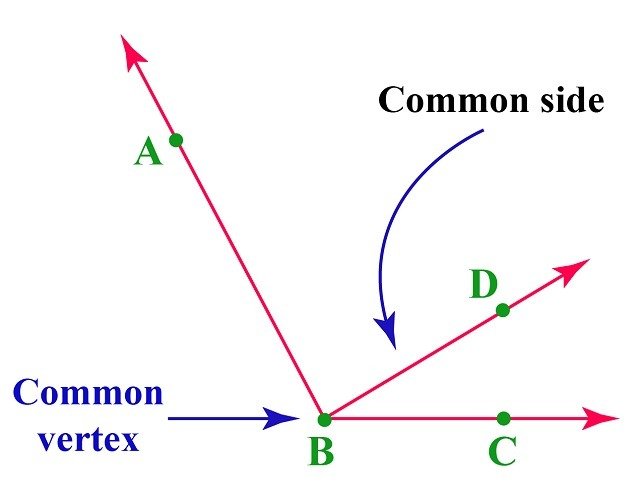
If two angle do not overlap and have a shared vertex and side, they are considered neighboring angle. To see what adjacent angle look like, look at the following illustration. Because Angle 1 and 2 share a vertex B and a side, BD, they are next to one other.

Definition of Adjacent Angles

Angle that are always positioned near to one another without ever overlapping are known as adjacent angle. They have a similar vertex and side.

Examples of Adjacent Angles

Numerous examples of adjacent angle in real life are visible.

In Actual Life, Adjacent Angle

The most common real-life example of adjacent angle can be seen in two pizza slices that are placed next to each other.

Another common example can be seen in the clock which shows the hour, minute, and second hand that form adjacent angle when all the 3 are away from each other.

We can find 3 adjacent angle in the steering wheel of a car.

corresponding angle – Linear Pair

A linear pair is a pair of neighboring angle whose measures add up to a straight angle. In a linear pair, the angle are additional.

Do parallel angles have the same side?

Adjacent angle have an arm in common. A common vertex unites adjacent angle. An adjacent angle does not cross over. On either side of the same arm, adjacent angle have non-common arms. There is not a common interior point between adjacent angle. The easiest way to recognize an adjacent angle is to look for the presence of a shared side and a common vertex.

Features of Neighboring Angles

The characteristics of adjacent angle are listed below to make their identification simple.

• Adjacent angle always share a common arm.
• They have a vertex in common.
• They don’t cross over.
• On each side of the common arm, they have a non-common arm.

The additional or complementary nature of two neighboring angle depends on the total of their separate measures.

How Are Adjacent Angles Found?

Two fundamental characteristics of neighboring angle make them easy to recognize: they always have a common side and a common vertex. Two angle will not be regarded as adjacent if they only meet one of these requirements. The angle must satisfy both of these requirements. Any two angle that have an angle between them yet share a same vertex, for instance, do not share a common side. They cannot therefore be neighboring angle. Examine the accompanying diagram to determine neighboring angle.

Important Information

A few key points about the nearby angles are listed here.

The angle created by two non-common arms and one common arm is the sum of the two adjacent angles.

The total of the adjacent angles created when a ray is in a straight line is 180°.

Two neighboring angle are referred to as a linear pair of angle if their sum equals 180°. Since the sum of the additional angle is 180°, all linear pairings are supplementary. All additional angle do not, however, have to be linear pairs. The lines must cross and create neighboring angle in order to make a linear pair.

The non-common arms form a line if the sum of the two neighboring angle equals 180°.

Conclusion

Angle that do not overlap and have a shared side and vertex are said to be adjacent. They are frequently encountered in daily life, such as in clock hands or pizza slices. Two neighboring angle form a linear pair when their sum equals 180°. Recognizing diverse angle relationships—a crucial idea in geometry—is made easier by having a solid understanding of nearby angle. Recall that adjacent angle have to share a vertex in addition to a side. If so, they are seen as being close together. grasp the fundamentals of geometry and how angle interact with one another in different shapes and structures requires a grasp of these angle.